What Are The Kinematic Formulas?

Kinematic Equations: The goal of this first unit of The Physics Classroom has been to investigate the variety of means by which the motion of objects can be described. The variety of representations that we have investigated includes verbal representations, pictorial representations, numerical representations, and graphical representations (position-time graphs and velocity-time graphs).

In Lesson 6, we will investigate the use of equations to describe and represent the motion of objects. These equations are known as kinematic equations.There are a variety of quantities associated with the motion of objects – displacement (and distance), velocity (and speed), acceleration, and time.

Knowledge of each of these quantities provides descriptive information about an object’s motion. For example, if a car is known to move with a constant velocity of 22.0 m/s, North for 12.0 seconds for a northward displacement of 264 meters, then the motion of the car is fully described. And if a second car is known to accelerate from a rest position with an eastward acceleration of 3.0 m/s² for a time of 8.0 seconds, providing a final velocity of 24 m/s, East and an eastward displacement of 96 meters, then the motion of this car is fully described.

These two statements provide a complete description of the motion of an object. However, such completeness is not always known. It is often the case that only a few parameters of an object’s motion are known, while the rest are unknown. For example, as you approach the stoplight, you might know that your car has a velocity of 22 m/s, East, and is capable of a skidding acceleration of 8.0 m/s², West.

However, you do not know the displacement that your car would experience if you were to slam on your brakes and skid to a stop; and you do not know the time required to skid to a stop. In such an instance as this, the unknown parameters can be determined using physics principles and mathematical equations (the kinematic equations)

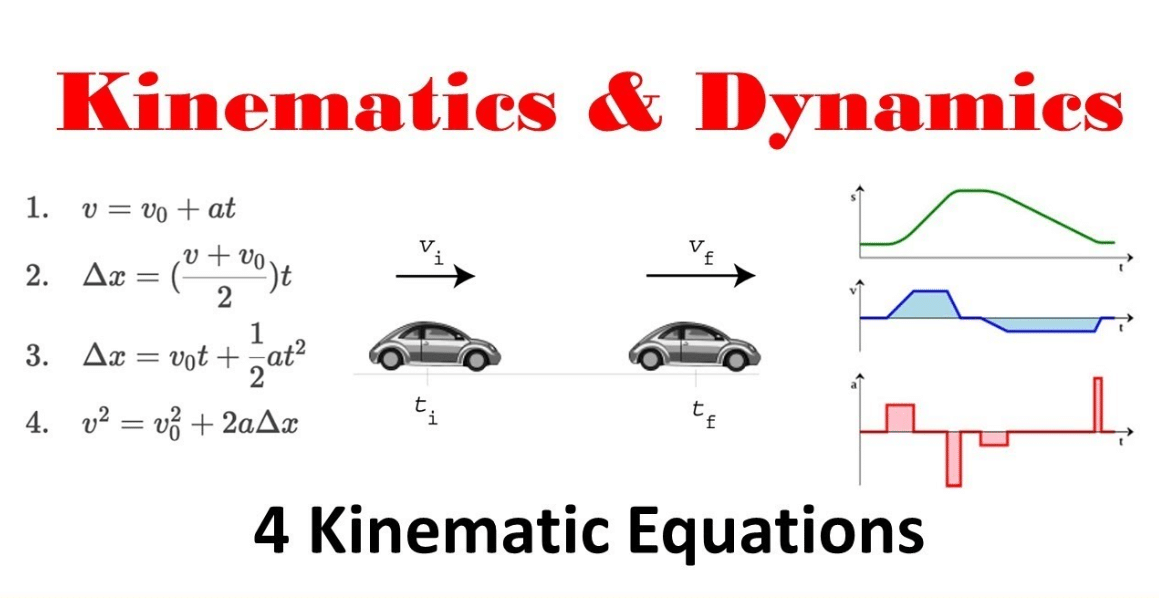
4 Kinematic Equations

Kinematics is the study of objects in motion and their inter-relationships. There are four (4) kinematic equations, which relate to displacement, D, velocity, v, time, t, and acceleration, a.

a) D = v_it + 1/2 at² b) (v_i +v_f)/2 = D/t

c) a = (v_f – v_i)/t d) v_f² = v_i² + 2aD

D = displacement

a = acceleration

t = time

v_f = final velocity

v_i = initial velocity

What are the 3 kinematic equations?

If we know three of these five kinematic variables— Δ x , t , v 0 , v , a \Delta x, t, v_0, v, a Δx,t,v0,v,adelta, x, comma, t, comma, v, start subscript, 0, end subscript, comma, v, comma, a—for an object under constant acceleration, we can use a kinematic formula, see below, to solve for one of the unknown variables.

How many kinematic equations are there?

four kinematic equations

The four kinematic equations that describe an object’s motion are: There are a variety of symbols used in the above equations. Each symbol has its own specific meaning. The symbol d stands for the displacement of the object.

What are the kinematic equations used for?

Kinematic equations can be used to calculate various aspects of motion such as velocity, acceleration, displacement, and time.

Kinematic Equations List

The kinematic formulas are a set of formulas that relate to the five kinematic variables listed below.

point, space, v, equals, v, start subscript, 0, end subscript, plus, a, t

point, space, delta, x, equals, left parenthesis, start fraction, v, plus, v, start subscript, 0, end subscript, divided by, 2, end fraction, right parenthesis, t

point, space, delta, x, equals, v, start subscript, 0, end subscript, t, plus, start fraction, 1, divided by, 2, end fraction, a, t, start superscript, 2, end superscript

point, space, v, start superscript, 2, end superscript, equals, v, start subscript, 0, end subscript, start superscript, 2, end superscript, plus, 2, a, delta, x

Since the kinematic formulas are only accurate if the acceleration is constant during the time interval considered, we have to be careful to not use them when the acceleration is changing. Also, the kinematic formulas assume all variables are referring to the same direction: horizontal x, vertical y, etc.

Angular Kinematic Equations

A freely flying object is defined as any object that is accelerating only due to the influence of gravity. We typically assume the effect of air resistance is small enough to ignore, which means any object that is dropped, thrown, or otherwise flying freely through the air is typically assumed to be a freely flying projectile with a constant downward acceleration of magnitude g, equal, 9, point, 81, start fraction, m, divided by, s, start superscript, 2, end superscript, end fraction.

This is both strange and lucky if we think about it. It’s strange since this means that a large boulder will accelerate downwards with the same acceleration as a small pebble, and if dropped from the same height, they would strike the ground at the same time.

[How can this be so?]

It’s lucky since we don’t need to know the mass of the projectile when solving kinematic formulas since the freely flying object will have the same magnitude of acceleration, g, equals, 9, point, 81, start fraction, m, divided by, s, start superscript, 2, end superscript, end fraction, no matter what mass it has—as long as air resistance is negligible.

Note that g, equals, 9, point, 81, start fraction, m, divided by, s, start superscript, 2, end superscript, end fraction is just the magnitude of the acceleration due to gravity. If upward is selected as positive, we must make the acceleration due to gravity negative a, start subscript, y, end subscript, equals, minus, 9, point, 81, start fraction, m, divided by, s, start superscript, 2, end superscript, end fraction for a projectile when we plug into the kinematic formulas.

Physics Kinematic Equations

The kinematic equations are a set of four equations that can be utilized to predict unknown information about an object’s motion if other information is known. The equations can be utilized for any motion that can be described as being either a constant velocity motion (an acceleration of 0 m/s/s) or a constant acceleration motion. They can never be used over any time period during which the acceleration is changing. Each of the kinematic equations includes four variables. If the values of three of the four variables are known, then the value of the fourth variable can be calculated. In this manner, the kinematic equations provide a useful means of predicting information about an object’s motion if other information is known.

For example, if the acceleration value and the initial and final velocity values of a skidding car are known, then the displacement of the car and the time can be predicted using the kinematic equations. Lesson 6 of this unit will focus on the use of the kinematic equations to predict the numerical values of unknown quantities for an object’s motion.

The four kinematic equations that describe an object’s motion are:

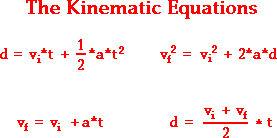
There are a variety of symbols used in the above equations. Each symbol has its own specific meaning. The symbol d stands for the displacement of the object. The symbol t stands for the time for which the object moved. The symbol stands for the acceleration of the object. And the symbol v stands for the velocity of the object; a subscript of i after the v (as in v_i) indicates that the velocity value is the initial velocity value and a subscript of f (as in v_f) indicates that the velocity value is the final velocity value.

Each of these four equations appropriately describes the mathematical relationship between the parameters of an object’s motion. As such, they can be used to predict unknown information about an object’s motion if other information is known. In the next part of Lesson 6, we will investigate the process of doing this.

Basic Kinematic Equations

Kinematics is the study of the motion of objects without concern for the forces causing the motion. These familiar equations allow students to analyze and predict the motion of objects, and students will continue to use these equations throughout their study of physics. A solid understanding of these equations and how to employ them to solve problems is essential for success in physics. This article is a purely mathematical exercise designed to provide a quick review of how the kinematics equations are derived using algebra.

This exercise references the diagram in Fig. 1, in which the x axis represents time and the y axis represents velocity. The diagonal line represents the motion of an object, with velocity changing at a constant rate. The shaded area (A₁ + A₂) represents the displacement of the object during the time interval between t₁ and t₂, during which the object increased velocity from v₁ to v₂.

This document will make use of the following variables:
v = the magnitude of the velocity of the object (meters per second, m/s)
v₁ = the magnitude of the initial velocity (meters per second, m/s) (in some texts this is vi or v₀)
v₂ = the magnitude of the final velocity (meters per second, m/s) (in some texts this is v_f)
a = the magnitude of the acceleration (in meters per second squared, m/s²)
s = the displacement vector, the magnitude of the displacement is the distance,
s = │s│ = d (vectors are indicated in bold; the same symbol not in bold represents the magnitude of the vector)
Δ indicates change, for example Δv = (v₂ –v₁)
t = time
t₁ = the initial time
t₂ = the final time